

We have studied the transport of magnetic fields by turbulent penetrative compressible convection. The interface dynamo model, working as it does by separating the regions of poloidal and toroidal field generation, relies on efficient mechanisms for transporting the toroidal field up from the overshoot region to the convection zone and then returning the weaker poloidal field to the overshoot region. Mean-field models have crudely parameterized this transport by a turbulent diffusion. Using the 3-D HPS code, we have conducted simulations that investigate realistic mechanisms for the transport of magnetic fields to and from this layer of convective overshoot. Magnetic fields in a stratified medium tend to be buoyant relative to their surroundings. A competition exists between this magnetic buoyancy instability (Cattaneo & Hughes 1988, Matthews et al. 1995) and the advective properties of the convective flows: we have investigated these transport mechanisms in a number of configurations.
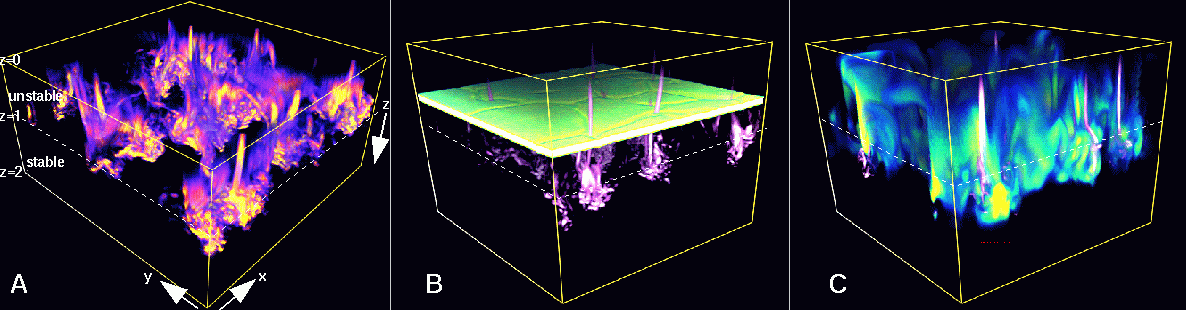
Firstly, we have taken a fully-developed solution for penetrative convection, imposed a layer of toroidal magnetic field in the convective region, and then studied the evolution of the magnetic field away from this initial condition (Figure 1). It has been shown previously that flux can indeed be expelled from a region of MHD turbulence into a quiescent region by turbulent eddies (Tao, Proctor & Weiss 1998), although the time-scale for the expulsion is too long to be efficient. A key question is whether turbulent convection can lead to expulsion of the field on a convective rather than a diffusive timescale. We find that entrainment of magnetic field by the strong convective downflows can produce a fast turbulent pumping mechanism, an example of which is shown in Figure 1, which exhibits volume renderings of the magnetic energy and enstrophy as the calculation progresses (Tobias, Brummell, Clune & Toomre 1998a). Figure 5b shows the initial configuration with the magnetic layer imposed at mid-depth in the convective layer. Some magnetic field initially rises due to magnetic buoyancy, but ultimately the magnetic layer begins to interact with the strong convection. The magnetic field is entrained by the local downdrafts (Figure 1c) and dragged down into the overshoot zone. These sinking plumes also amplify the fields locally by stretching. The net result of both the advection and the local amplification is a transport of magnetic energy and flux from the convection zone into the overshoot region, where it can be stored. The competing effect of magnetic buoyancy means that some flux is lost through the top of the computational domain. However a significant amount of flux is still expelled from the convection zone into the overshoot region. The rate of this expulsion is not sensitive to the strength of the initially imposed magnetic field. In these turbulent simulations the convective flows are sufficiently strong that the field is advected in a surprisingly passive manner.

Secondly, we have imposed a layer of toroidal magnetic field in the overshoot region, and then studied the evolution (Figure 2) of the magnetic field away from this initial condition (Tobias, Brummell, Clune \& Toomre 1998b). Under the action of magnetic buoyancy, the field initially rises into the convection zone and then quickly breaks up to form a fibril structure where the field varies on small scales (see flux tube structures in the magnetic field in Figure 2c). Whether the field continues to rise or is successfully held down in the overshoot region by the flow of the downward plumes (turbulent pumping effect) depends critically on the magnetic field strength. If the field is strong then the buoyancy instability manages to move flux from the overshoot region into the convection zone; if the field is weak then the flux may be trapped in the overshoot region. A strong field is one whose magnetic energy is comparable to the kinetic energy in a downflow structure. This competition can act as a selection mechanism ensuring that only the strongest field escapes from the overshoot region through the convection zone, leaving the rest of the field to continue to be amplified by the shear until it reaches strengths large enough so that escape is possible.
Our studies show that this crucial component of the interface dynamo does indeed operate in an efficient manner. Since the flux is pumped and locally stretched by downward plumes, the field has a tendency to align itself with a preferred orientation. Once this field has reached the overshoot region, it may be amplified by the large radial shear of the tachocline into a strong toroidal field. The fact that the field has a large component aligned with the downflows when it is pumped may be important for the formation of a strong coherent toroidal field with a preferred direction in the stable region; this needs to be confirmed by further simulations. Moreover, the turbulence may also confine this large toroidal field, with our studies in (Figure 2) suggesting that only the strongest field rises and the majority is pinned in the overshoot region. Strong magnetic field can form compact structures, either via interactions with the overshooting convection or by instabilities (Matthews et al. 1995), and subsequently rise, although the layer as a whole may be contained. In the tachocline, the field may continue to be sheared until it reaches a critical strength that allows flux to escape from the overshoot region. Such a mechanism would select strong fields in isolated structures to emerge at the solar surface.
This page prepared by Nic Brummell, Laboratory for Computational Dynamics, University of Colorado.